(Ed. This is part 5 of a multi-part series. Comments that indicate a failure to read previous entries shall be mocked and, possibly, moderated with extreme prejudice. The author took the time to do the research, you can take the time to read it)
(Part 1 Part 2 Part 3 Part 4)
What Causes Concussions:
As we discussed in article 1, the primary cause of concussions in SCA combat and in HEMA is high levels of acceleration of the head. However, the way that many fighters envision concussions– that they occur because the brain sloshes around and strikes the inside of the skull– is generally incorrect, as this kind of concussion requires far more force than we are using (these require vehicular accident-level forces). Instead, we are almost exclusively dealing with concussions that result from diffuse axonal injury.

The location of an axon in a brain cell (neuron). Image from: http://www.neuroscientificallychallenged.com/glossary/axon/
Diffuse axonal injury is mainly the result of rotational acceleration of the head because this kind of motion creates shearing forces that cut and damage axons throughout the brain. Axons are one of the main parts of brain cells (neurons) and they form long fibers that carry the signal from one brain cell to another. Outside of the brain, axons are what form nerves and most of the spinal cord, and as you can imagine, cutting axons inside the brain is bad. When a neuron has its axon cut or damaged, it can no longer send messages like it is supposed to, which can trigger a neuron to kill itself. Furthermore, this damage creates an inflammatory response that causes other types of damage to the brain and is largely responsible for the symptoms that follow concussions (e.g. tiredness, confusion, memory problems, fuzziness, problems with attention).
Because this is the kind of concussion that we are most likely to receive from SCA combat, we know that any attempt to protect ourselves from concussions must prevent rotational acceleration of the head. In order to see how this works, we must consider what happens when two objects collide.
In physics, when two objects interact, they do so by exerting something called force. Essentially, force is a measurement of the push or pull that one object exerts on the other (or both objects exert on each other). We know from Newton’s First Law of Motion that force must be applied to an object in order to cause it to accelerate. Likewise, we know from Newton’s Second Law of Motion that force is related to acceleration as a consequence of inertia, that is, an object’s tendency to remain stationary or its tendency to continue moving with constant velocity.
To put it another way, inertia is an object’s ability to resist acceleration and force is what causes that acceleration. When we are considering acceleration through space (translational acceleration), an object’s inertia is the same as its mass. However, when we are considering rotational acceleration, we must instead calculate something called a moment of inertia, which takes into account the fact that when an object rotates, not all of its mass is undergoing the same amount of acceleration. Because of this, Newton’s Second Law of Motion provides us with two different equations:
Force = mass * translational acceleration or F = m*a
Torque (τ) = moment of inertia (I) * rotational acceleration (α) or τ = I*α
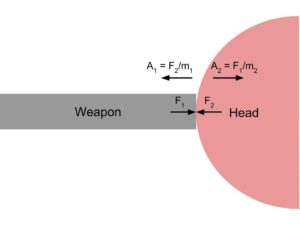
To put this another way, when a weapon strikes someone’s head, that weapon exerts force on the head and likewise the head resists this force based on its inertia. Since we also know that for every action, there is an equal and opposite reaction (i.e. Newton’s Third Law of Motion), we know that the head also applies an equal amount of force to the weapon (i.e. in the opposite direction). This means that both the weapon and the head will undergo acceleration relative to their respective inertias following a collision because, importantly, force is not conserved in the same way that energy and momentum are conserved. We can see this in the following figure:
Here, F1 is the amount of force applied by the weapon and F2 is the force of resistance provided by the head. Due to Newton’s Third Law of Motion, we know that F2 = -F1, where the negative sign indicates that F2 occurs in the opposite direction from F1. We also know that the acceleration of the head, A2 is the force F1 divided by the mass of the head, m2 due to Newton’s Second Law of Motion. Therefore we know that it is force that causes the head to accelerate.
The blows that cause concussions:
As noted in article 1, the amount of acceleration necessary to cause a concussion is at least 60Gs. This is a fairly high value, so let me be clear, causing a concussion requires you to hit someone with a lot of force.
Because we know that force is dependent on mass and acceleration, we should expect that strikes that place a person’s body mass behind the blow and/or provide a lot of acceleration are the strikes that are most likely to cause concussions. There are a number of techniques that fulfill these requirements, however for the most part, such techniques will fall into one of the following categories:

This is going to have way more than 22lbs of force!
Image from: https://www.facebook.com/photo.php?fbid=10208676965068728&set=t.752572217&type=3&theater
- Jumping/falling – One of the easiest ways to hit someone hard is to “jump” upwards during your attack such that you are falling when you strike your opponent. These strikes are going to always hit with an amount of force equal to a fighter’s body weight. An extreme example is pictured below. Because Wistric has jumped upwards, if he lands a strike while he is falling, he will hit his opponent with the full ~250lbs of his body weight. This is obviously far more than the 22lb typical blow that Llwyd’s machine recorded (Article 2). Perhaps even more importantly, as long as Wistric’s sword strikes his opponent before his feet hit the ground, there’s absolutely nothing Wistric will be able to do in order to reduce this force. Wistric is also at serious risk of being hit even harder by his opponent, as they may use any of the other techniques here to add their own body mass + muscle strength to the blow. It is also important to keep in mind that this occurs even during far less obvious examples of “jumping.” Any strike where one fighter throws their body forward or upwards, even if it’s only a few inches, runs the exact same risk.
- Punching – Professional boxers punch with around 1000 lbs of force, and while the number of us who are professional boxers is near-zero, that doesn’t mean that our punches are anywhere near as gentle as the force levels recorded by Llwyd’s machine. Punching techniques achieve these force levels by engaging core and leg muscles and by coupling the body mass behind the arm by extending it during the strike. As a result, these blows couple the body mass with significant levels of acceleration in order to generate a lot of force.
- Kinetic Linking – The kinetic linking used in SCA rattan combat is similar to delivering a punch in many ways, however when these body mechanics are used to perform a cut, they produce a “whipping” action with the sword that can produce extreme levels of acceleration. Swinging a sword is similar in some respects to swinging a baseball bat and adult baseball players frequently have a bat speed in the 70-80 mph range, which is more than 30 m/s. This speed is reached in around a second (if not less), so acceleration of ~30m/s2 is probably a reasonable (if not low) estimate of the acceleration of the weapon. When this is coupled with the mass of a body, it becomes possible to generate an amount of force that is roughly 3x someone’s body weight or more. The key limitation to this ability is that as the point of impact is moved further down the weapon away from the person delivering the strike, it becomes more difficult to place one’s body mass behind the blow (because of the leverage disadvantage). This means that “short stick” strikes are particularly dangerous as they couple a punch with an acceleration advantage provided by the weapon’s leverage.
So, why aren’t we Always getting Concussions?
Once again, we are running into a limitation in the way that we have modelled the impact. In reality, the person receiving the blow is also able to put their body mass into resisting the strike. They do this by engaging their neck muscles (which are stronger than you might expect). If the person receiving the blow does this successfully, then they will dramatically increase the amount of resistance (i.e. the effective mass) of the head against acceleration, which will make it difficult for a fighter to be concussed by a blow that they were prepared to receive.
We can see this play out in other sports. For instance, one of the more interesting findings has been that one of the best methods for reducing concussions has been the introduction of helmet-less tackle drills(Myers et al., 2015), which are thought to train players to avoid head impacts and improve their ability to prepare to take a hit. Being prepared to be struck is also important in other sports like boxing or mixed martial arts. If you watch the following video carefully, you’ll see that the knock-out blows happen when the recipient is unprepared to be struck. More specifically, you’ll see that they occur during moments where the recipient is pulling their head away from the blow, which makes it impossible to actively resist.
A dramatic disparity in body mass/strength is the main limitation to the notion that it is difficult to concuss a fighter who is prepared to receive a blow. Sometimes one fighter is capable of exerting a significantly greater ratio of force than the smaller fighter can resist, but such a blow would clearly be excessive, even on the SCA armored field. However sometimes accidents happen, which is one of the reasons why technique and conditioning are important as discussed in article 4. This is also a large part of why weight classes exist in other combat sports and why neck strength conditioning is considered to be very important in sports like football, boxing, and wrestling.
The ability to resist impacts to the head with your musculature is the primary means of protection against brain injuries.
We should also avoid coming to the conclusion that if a concussion occurs, it is the recipient who was at fault. It isn’t possible for fighters to be ready to take every hit that they are struck by. This ability is improved with skill, practice, etc, but even in the video above, we see professional fighters caught unprepared.
Ultimately, this means that concussion protection largely boils down to not hitting too hard, particularly when your opponent is unaware/unprepared to be struck.
What about a Helmet?
Helmets are relevant to protecting against concussions only when the recipient is unprepared, because, as we have just seen, it is really hard to concuss someone who is prepared to receive a blow. Helmets are therefore a form of passive protection for instances where a fighter is unprepared to be struck. Unfortunately, concussion protection is in its relative infancy. It is only in the last few years that the consequences of concussions have been seen as anything more than a temporary inconvenience and so preventing brain injuries is not something that most protective equipment has been designed to do. Instead of reducing the acceleration of the head, helmets instead are designed to minimize pressure by spreading impacts out over a greater area. This is useful for preventing pain, bruising, pressure cuts, facial bone/skull fracture, eye/ear damage, hearing loss, and other soft-tissue injuries. However, helmets do not generally spread an impact out over a greater portion of the body than the head. This means that they provide nearly zero effective protection against the acceleration of the head. In other words, if something is only attached to the head, it can’t spread an impact out over more than the head. Despite this, the SCA’s traditional wisdom seems to believe that either the mass of the helmet or the padding itself will protect against concussions. However, as will be shown, neither of these provide meaningful protection against concussions.
Mass:
One of the key ways in which a helmet can provide protection to the head is by being heavy. Newton’s Second Law of Motion tells us that the inertia of the head is crucial in determining the amount of acceleration that either undergoes and so clearly adding mass to the head provides some degree of additional protection. However, the mass of even a very heavy helmet is still a full order of magnitude smaller than the mass of your opponent behind their weapon.
Furthermore, since we expect that rotational acceleration is the key to concussions, we also need to understand that the effects of added mass on the moment of inertia are greatly diminished for relatively small objects such as the human head. For instance, the moment of inertia of a sphere is calculated using the equation:
I = ⅖ * mass * radius2
In this equation, radius is measured in meters, and so for an object such as the human head that has a radius of ~10 cm, the effect of mass is multiplied by 0.01 * 0.4 = 0.004. However, it is also worth noting that when calculating rotational acceleration, the acceleration is measured in terms of radians/s2 rather than in meters/s2, so in order to compare this directly with the acceleration threshold of 60Gs, we must convert our acceleration from radians to meters by multiplying the angular acceleration by the radius (i.e. dividing by 10) and so we know that the effect of mass is essentially multiplied by 0.0004 when we are considering its effect on resisting rotation.
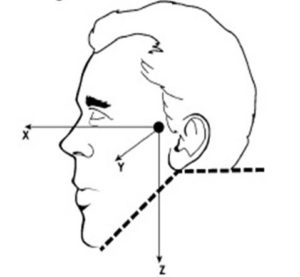
While a sphere might be an acceptable model of moment of inertia, we should keep in mind that the head is not a sphere. Calculating the moment of inertia for an irregularly shaped object like the head can be tricky, but fortunately there are a number of researchers who have figured this out for us. Yoganandan et al. (2009) provides a summary of multiple studies that have calculated the moment of inertia of the human head for rotation around the x (left-right tilt), y (up-down rotation), and z (left-right rotation) axes using human cadavers.
In order to find suitable values, I averaged the values found in tables 19 and 20, resulting in the following mass and moments of inertia:
Mass: 4.17 kg
Ix = 0.0186 kg-m2
Iy = 0.0215 kg-m2
Iz = 0.0168 kg-m2
In order to calculate the amount of resistance against rotation that added mass provides along each of these axes, we must divide by the average mass:
Ix/kg = 0.0186 kg-m2/4.17 kg = 0.0045 I/kg
Iy/kg = 0.0215 kg-m2/4.17 kg = 0.0052 I/kg
Iz/kg = 0.0168 kg-m2/4.17 kg = 0.0040 I/kg
If we were to consider the effect of a very heavy 10kg helmet and 4kg head, we would need to multiply that 14kg by about 0.0045 in order to figure out the amount of resistance this would provide against the force of an impact (0.063kg). However, in order to see how this relates to our 60G threshold, we must also convert from radians to meters as we did before by dividing the mass of the weapon that we used in the calculator by 10 (because the head has a radius of ~10cm). So we have F = 14kg * 0.0045 * 600m/s2 /0.1m = 378 N = 85lbs.
If we compare that against the head by itself, F = 4kg * 0.0045 * 600m/s2/0.1m = 108 N = 24 lbs. That 50 lb difference may seem like quite a bit of protection if we compare against the amounts of force delivered in article 2, however those blows probably underestimate the amount of force in a typical blow and certainly do not reflect the types of blows that lead to concussions. Likewise, 10kg is heavier than almost every helmet on the rattan field. Given that concussions are caused by shots that have your opponent’s body mass behind them, we need to resist several hundreds of pounds of force in order to protect against concussions. Against those numbers, 50lbs is completely insufficient to be relied upon for protection and so we cannot rely on a heavy helm to protect us.
That isn’t to say that there might be a few shots where a heavy helm makes the difference between a concussion and no concussion, which might be enough to cause fighters to choose to wear heavier helmets as a personal choice, but the notion that a heavy helm can be relied on to prevent concussions or that a fighter who receives a concussion received one because their helmet wasn’t heavy enough is patently false.
Padding and Suspension Systems:
Aside from mass, the other aspect of helmets that many focus on as a way of preventing concussions is the padding/suspension system that it provides. Here we will address the reasons why neither padding nor suspension systems protect the head from acceleration.
Please keep in mind that the following discussion is focused solely on the protective effects of padding against concussions (i.e. its ability to prevent acceleration of the head). This article does not make the claim that padding provides no benefit; in fact the opposite is true. However, the benefits of padding are limited to reducing pressure. Pressure can cause several different types of injuries to the head (including skull fracture) and is the only factor that influences pain and discomfort, but do not mistake an absence of pain for an absence of acceleration. The experience of pain is separate from the mechanisms that cause concussions.
Padding and Suspension Systems are the Same Thing:
Before we get started, it is also important to understand that padding and suspension systems are functionally equivalent to each other and are also functionally equivalent to springs. In practice, padding is a compression spring and suspension systems are expansion springs, however all springs are governed by the same mathematical functions. We know that padding/suspension systems are functionally springs because:
- Padding, suspension systems, and springs all provide progressive resistance to force based on how far they have been compressed
- All three have sufficient elasticity to return to shape after they are released
- All three convert kinetic energy to potential energy and and potential energy to kinetic energy
- the materials used to construct padding and suspension harnesses are themselves springs (i.e. the fibers that make up cloth and batting and the air bubbles in foam are themselves tiny springs).
- Padding/suspension system return to their original shape
Padding does not affect the amount of Force experienced by the head:
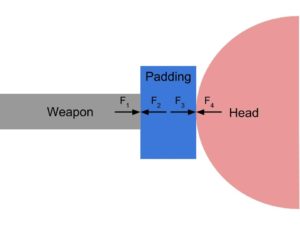
Consider the illustration provided above. As before, when the weapon strikes, it applies some amount of force, F1 to the padding. As a result of Newton’s Third Law of Motion, we therefore know that F2 = -F1 just like before. If this level of force exceeds the inertia of the padding, then the padding will begin to accelerate towards the head. Now, the reason that it is important that padding functions like a spring is that this allows the front surface (the side closest to the weapon) to accelerate separately from the back surface (the side closest to the head). When this happens, the padding is compressed and the distance between the two surfaces becomes closer by some amount d– the displacement. We also know that according to Hooke’s law, the amount of force required to compress the padding is calculated using the equation F = -k*d where k is the spring constant that describes how stiff the padding is. The materials that are used to pad SCA helmets have relatively low k values, generally speaking, but that’s not particularly important to understanding why padding doesn’t prevent acceleration of the head.
When the padding compresses (due to F1), Hooke’s Law tells us that a linearly increasing amount of force is required to compress the padding further. However, for any given moment of time, the padding itself will be attempting to expand by exerting an equal amount of force in both directions. This means that regardless of the amount of compression, the force on the front side of the padding, F2 will always be equal in magnitude (and opposite in direction) to the force on the other side of the padding, F3, or expressed mathematically F2 = -F3.
Given that we know that F1 = -F2 and we know that F2 = -F3, then we know that it is always the case that F1 = F3 and therefore padding has zero effect on the force experienced by the head nor the acceleration of the head.
The Padding Prevent the Head from Moving:
While the above explanation is by itself sufficient to demonstrate that padding has no effect on the force applied to the head from an impact, there will be some who remain unconvinced, and so let me offer an alternative explanation for why padding doesn’t help.
In order for padding to compress, it must have something to push against.
This effect forms the basis of the classic “falling elevator” problem from high school physics. The scenario is as follows: You are standing on a scale on an elevator. When the elevator is stationary, the scale tells you your weight (which is a measurement of force, not mass).
When the elevator goes up, the measurement of your weight will increase because the elevator is applying force upwards that exceeds gravity, causing it to accelerate towards you, which causes the springs to compressed more (which is dependent on the resistance you provide, i.e. your inertia/mass).
When the elevator goes down, the measurement of your weight will decrease because the upwards force applied by the elevator is less than gravity and so the elevator is accelerating away from you. This means that the springs are compressed less, which is what results in the lower weight measurement.
The problem is described in the following video:
So what happens when the elevator is in free-fall? The weight measurement becomes zero.
The falling elevator problem is analogous to the problem posed by adding padding to our helmets. The weapon striking the helmet is the equivalent of gravity in the above scenario while the force applied by the elevator is equivalent to the resistance provided by the head. We can therefore see that when the head is accelerated due to an impact, that the padding doesn’t compress because the head is moving away from it and provides no resistance.
Does Padding “Absorb” the Blow?
There are many who argue that padding reduces force by “absorbing” a blow by compressing. In fact, this argument is incorrect for two reasons: first, it confuses force with energy and second, it fails to understand that the padding won’t compress much during impacts that are likely to cause concussions.
Energy and force are certainly related concepts, however energy is defined as the ability to do work and likewise work is defined as the exertion of force over some distance. When you stand on the ground, your body is exerting force (your mass * acceleration due to gravity) but, since you are not moving, you aren’t doing any work and therefore your energy is zero. If you instead stood on a chair, you’d still be exerting the same amount of force (on the chair). You also wouldn’t be doing any work but your position on the chair does represent your ability to do work and therefore you would have an amount of (potential) energy related to your mass, the acceleration due to gravity, and the height of the chair. One of the key aspects of springs is that they convert kinetic energy (KE) into potential energy (PE) when they are compressed. However this conversion is a consequence of displacement. Hooke’s law tells us that the force (F) required to compress a spring is given by the equation F = -k*d, where k tells us how stiff the padding is (i.e. spring constant) and d is the displacement distance. Importantly, this amount of force is also the amount of force applied by padding that is compressed a given distance and this amount of force is exerted in both directions as I pointed out earlier.
Determining whether a spring (or our padding) will compress is largely a matter of exerting sufficient force to exceed its resistance at a given amount of displacement. In order for this to be possible, the forces applied to both ends of the spring must exceed this value (or the spring will simply accelerate through space rather than compress). When the padding is compressed, it temporarily stores an amount of energy according to the equation PE = 1/2 k * d2. In practical terms, this means that the padding in our helmets will only compress up until the point where the needed force to continue compressing the spring exceeds the resistance provided by either the head or weapon. This creates a finite set of possibilities:
- The padding is stiffer than the resistance provided by the head and does not compress at all before the head accelerates as if the padding were not present.
- The padding is soft enough to compress, but compresses to the point where the force to continue compressing the padding exceeds the resistance of the head, at which point he head accelerates as if the padding were not present.
- The padding is much softer than the resistance provided by the head and therefore it fully compresses, at which point he head accelerates as if the padding were not present.
If case 1 above is true, then the padding has behaved no differently from a solid object, and so let’s look at cases 2 and 3. As noted above, we specifically need a helmet to protect us is when our head isn’t providing much resistance. Therefore, if case 2 is correct, then we should expect a very small amount of compression, which means that the padding isn’t storing much energy. Likewise, if case 3 is correct, then the k-value of the padding must be very low and so again, the padding isn’t storing much energy. In all three cases, there is a point in the impact where the padding behaves as if it is not present. Furthermore, we can see that the padding’s ability to store energy is directly related to displacement rather than force, and finally, we can see that in all cases, the amount of energy stored by the padding is zero or near zero. That is to say that when we are most at risk for a concussion, the padding doesn’t help.
Does Padding Serve as an Early Warning System?
As mentioned above, the key factor in preventing your head from accelerating is the engagement of your neck musculature. This requires that you either keep your neck engaged at all times (as was suggested by article 4) or be aware of incoming blows and respond accordingly. Ultimately the entire point of a passive protection system is for the times that you fail to do this. In this regard, the fact that padding applies force as soon as it is struck, and that this force is smaller (i.e. only the spring constant of the padding itself), would suggest that ample padding might provide an additional tactile warning system for when vision has failed.
In order for this to work, the padding must allow sufficient time for a response to occur. Human response times to tactile stimuli are in the 150-200 ms range, so that’s how much of an early warning padding would need to give us in order to help us.
It would be nice to have some high-speed camera footage for measuring this; however we can make a good estimate by measuring the length and duration of a fencing lunge. According to Gholipour et al. (2008), a modern fencing lunge covers approximately 1 meter in 1.5 seconds.
Importantly, as noted above, the amount of compression will be negligible and so this won’t really slow down the lunge. While more force is required to compress the padding, blows that can cause concussions already must provide a lot of force, so we shouldn’t expect this to matter. If we assume for the sake of a “best-case” scenario with relatively thick padding that is approximately 5 cm (2”) thick, then we can calculate the amount of time necessary for the lunge to cross this distance as 75 ms. We can also then determine that for padding to provide an early warning system, we would need at least 6” of padding.
However, we must also keep in mind that padding, like a spring, cannot compress fully and so there will be some remaining thickness which will cause the head to be struck earlier than if there was not any padding, thus reducing a fencer’s ability to respond to visually seeing that they are about to be struck. However, given that the lunge is several times slower than the ability to respond to visual stimuli (~200-300ms), we likely should expect that this effect is negligible.
The Dangers of Armoring Up:
While the analysis provided above may suggest that we can simply strap large quantities of lead weights to our heads and then hit each other as hard as we like, there are practical and safety limits to the amount of mass that can be added to the head. There is even some evidence in other sports that increased head protection can lead to more concussions. For instance, head gear was eliminated from men’s boxing in 2016 due to the fact that it increased the risk of concussions. This Wired article provides a more detailed explanation. There are ultimately a number of ways that increasing the armor we place on our heads can put us in more danger.
Bigger helmets = more head hits
Perhaps the most important consideration is that by adding mass and padding to our helmets, we make them larger. This creates a larger target, which makes head shots more likely.
Adding too much mass to the helmet puts you at substantial risk for whiplash and can increase your risk of spinal injury
Whiplash is an umbrella term for tears of the ligaments of the spine, tears in neck muscles, and damage to cervical vertebrae that occurs when the neck is hyper-extended (stretched out too much). Adding too much mass to the head puts you at substantial risk of this occurring because it can result in situations where the neck muscles are incapable of stopping the momentum of the combined head and helmet. In extreme cases, this may be sufficient to break your neck. Furthermore, if the purpose of adding mass to the helmet is to prevent concussions when the neck muscles have already failed to engage, then this mass is coming into play precisely when the neck muscles have failed to engage.
Situations that can lead to whiplash injuries include times where the head is moving and the body is not, such as collisions between fencers, falls (especially when falling onto something that stops part of the body and allows the head to continue to move… like a hay bale or another fighter), and in HEMA, grappling/throws.
Increased Mass and/or Padding may lead to Increased Calibration
Increased helmet mass and/or padding will reduce the perception of pain/pressure that result from being struck. This lack of sensitivity may cause fighters to ignore blows that they would have taken if not for their armor, which can cause their opponent to hit them harder. Alternatively given the absence of perceived pain/pressure/motion from hard blows, fencers may be encouraged in their belief that hitting hard is safe because they are wearing armor despite the fact that the sensation of pain and pressure are completely independent of the mechanisms that cause concussions.

Light!
Image From: https://www.facebook.com/photo.php?fbid=10153001416096776&set=t.752572217&type=3&theater
Increased Mass and/or padding may Cause you to Fail to Notice that you have been Concussed
The brain does not have any pain/pressure sensors inside of it, so the presence or absence of pain doesn’t tell us whether we have sustained a brain injury, but impacts that can cause a concussion typically hurt. Helmets do a good job of mitigating pain, but as shown above, do not mitigate acceleration to the same extent. As a result of this, heavier and/or more padded helmets can make it harder to tell whether you have been concussed.

It is absolutely vital that fencers who have been concussed be removed from the field as secondary impacts are known to have a major impact on the severity of a concussion and its permanent neurological effects. Furthermore, these secondary impacts do not need to be nearly as hard as the initial injury, so even relatively soft impacts can cause life-altering permanent damage following the initial injury. Because of this, efforts to alleviate the effects of concussions in other sports have focused on improving the ability for coaches, staff, and players to recognize concussions and on building policies that require injured players to be removed from the field. Interfering with a fencer’s ability to recognize a concussion is the worst thing that we can do when attempting to manage concussion risk.
Increased Armor = Increased Mass = Harder Hits:
As noted above, the strikes that are the most risky for causing a concussion are those that combine body mass with acceleration. If fighters increase the amount of armor that they are wearing, they are adding to the mass of their body. If we consider the force that results from a jumping/falling strike, for instance, we can see that adding the mass of a heavy helmet (10 kg as in our earlier example) would increase the force of a blow by around 22 lbs. (10kg * 9.8 m/s2 = 98 N = 22lbs), which nearly halves the effective protection provided by their opponent’s helmet. If we also consider that harder hits will result in fighters wearing additional armor on the rest of their body, the the added mass behind strikes may fully negate the protection provided by the armor in the first place. Therefore, a person who armors up to protect themself, is in fact becoming more of a danger to their opponent. If everyone armors up to the same degree, the equipment becomes progressively more ungainly and expensive to no net benefit.
The Dangers of Misinformation:
Recently the SCA’s marshallate has taken the stance that increased padding will provide protection against concussions. While it is reasonable to expect that helmets (and fencing masks) be sufficiently padded and be in good condition, we have demonstrated here that the padding does not protect against concussions. This kind of misinformation makes us all less safe.
Legal Liability:
Participants in SCA combat sign a waiver that indicates that they understand that there are risks associated with participation. However, these waivers rely on the assumption that participants are providing informed consent. When marshals acting on behalf of the organization and more importantly, the society marshals spread misinformation, they are removing participants’ ability to be informed. Furthermore, if it can be demonstrated that these officers should have known otherwise, then it likely exposes the organization to a lawsuit on the basis of negligence if a participant receives a concussion despite the padding in their helmet.
Increased Calibration:
Misinformation, especially misinformation spread by the marshallate’s office may also encourage fighters to feel that hitting harder is safer than it actually is and so it may also be a factor in increasing calibration.
Failure to Recognize Concussions:
Similarly fighters and marshals who are acting on the false belief that increased padding or mass will provide protection against concussions may be less likely to consider that a concussion has occurred and may therefore be less likely to recognize when one does occur. As noted above, failure to remove fighters from the field immediately following a concussion creates a significant danger of permanent brain damage.
Excuses Bad Behavior:
One of the most dangerous effects of the false belief that armor prevents concussions is that it provides an excuse for fighters who are a danger to themselves and others on the field. As we have shown here, helmets cannot be relied upon to protect against concussions and so it is patently false that if a concussion occurs, that it occurred due to armor failure or a lack of padding. In fact, it was precisely this kind of ruling that motivated me to write about concussions in the first place.
Other Concerns:
In addition to the ways that the false belief that helmets protect us from concussions make SCA combat less safe, there are other problems that this false belief creates.
Unnecessarily Complicated rules:
If fencing masks are going to continue to be legal armor, then creating a separate set of requirements for helmets that cause them to provide a greater level of protection creates a double-standard in the rules and likewise creates an illusion that it is ok to increase calibration when people are wearing helmets.
Cost:
Acquiring a set of equipment isn’t cheap and adding to the required equipment should not be taken lightly. With 2000 authorized fighters, even the addition of a $50 piece of equipment represents an economic cost of $100,000 for our membership. Furthermore, any increased cost will remove some fighters from being able to participate.
Equipment damage:
If calibration increases as a result of increased armor, the wear and tear on equipment will represent an additional cost for participation and will also create an increased risk from broken blades, etc.
Sexism/ableism:
The requirement or practical necessity for increased armor will exclude participants who are smaller in stature or who have less physical strength.
In Conclusion:
Helmets do not provide protection from concussions because they do not prevent the head from accelerating. While increased mass does have some protective effect, the effect is not sufficient to eliminate the risk of concussions that occur due to the types of blows that are most likely to produce concussions in the situations where concussions are most likely to occur. Padding or suspension systems cannot reduce the acceleration of the head because they do not alter the amount of force delivered to the head. Increasing the amount of armor in an effort to prevent concussions is likely futile and may increase the risk for concussions and/or other serious injuries.
Most importantly, the marshallate has an important role in preventing concussions. It is vital that marshals understand that armor cannot prevent concussions and that recent statements regarding padding in helmets be revised because this sort of misinformation poses a hazard to the safety of SCA combattants.
References:
- Yoganandan, F.A. Pintar, J. Zhang, J.L. Baisden. Physical properties of the human head: mass, center of gravity and moment of inertia. J. Biomech., 42 (9) (2009), pp. 1177–1192 (PMID: 19428013)http://www.sciencedirect.com/science/article/pii/S0021929009001444
- Guskiewicz KM, Mihalik JP, Shankar V, et al. (2007). “Measurement of head impacts in collegiate football players: Relationship between head impact biomechanics and acute clinical outcome after concussion”. Neurosurgery 61 (6): 1244–52; discussion 1252–3.doi:10.1227/01.neu.0000306103.68635.1a.
- Myers, JL. et al. Early Results of a Helmetless-Tackling Intervention to Decrease Head Impacts in Football Players. Journal of Athletic Training, December 2015 DOI: 10.4085/1062-6050-51.1.06
- Gholipour, M., Tabrizi, A., and Farahmand, F. Kinematics Analysis of Lunge Fencing Using Stereophotogrametry. World Journal of Sport Sciences(2008), 1(1): 32-37.
- Guskiewicz KM, McCrea M, Marshall SW, et al. Cumulative Effects Associated With Recurrent Concussion in Collegiate Football Players: The NCAA Concussion Study.JAMA. 2003;290(19):2549-2555. doi:10.1001/jama.290.19.2549.
- R.C. Cantu. Guidelines for return to contact sport after a cerebral concussion. Phys Sports Med, 14 (1986), pp. 75–83
Pingback: Tibbie Crosier
Pingback: Gawin
Pingback: Indy
Pingback: Indy
Pingback: Gawin
Pingback: Tibbie Crosier
Pingback: Gawin
Pingback: Tibbie Crosier
Pingback: Gawin
Pingback: Dante di Pietro
Pingback: Tibbie Crosier
Pingback: Dante di Pietro
Pingback: Ken Mondschein
Pingback: Gawin
Pingback: Siegfried Outlands
Pingback: Dante di Pietro
Pingback: Tibbie Crosier
Pingback: Orlando Cavalcanti
Pingback: Gawin
Pingback: Orlando Cavalcanti
Pingback: Orlando Cavalcanti
Pingback: Mf